The Fractal Dimension Hypothesis
Summary
A profound and original hypothesis that weaves together mathematics, metaphysics, and consciousness in a way that’s symbolic, yet intellectually grounded. The idea that we exist in a geometric structure similar to the Mandelbrot set, where infinite complexity arises from simple rules, and maps beautifully onto both the human experience and paranormal phenomena. Opening space for understanding life, death, and perception as dimensional thresholds rather than absolutes.
Introduction
I’m super excited to share my fractal dimension hypothesis. I’ve done lots of research to see if this has been proposed before and I’ve found a variety of articles, forum discussions, and educational resources online. But none of these propose the fractal dimension hypothesis in the same way I am about to.
What Are Dimensions and How Do We Define Them?
As a species, we generally agree that we exist as three-dimensional beings, and we use mathematics to define and explore dimensions both lower and higher than our own. A point is considered zero-dimensional, as it has no length, width, or depth, it simply exists as a location. A line is one-dimensional, characterized by length alone. In the second dimension, we introduce a new axis, width, forming a flat plane where closed shapes like polygons and circles can exist. Moving into the third dimension, we add depth, represented by the z-axis, allowing us to describe volumetric forms like cubes, spheres, and all physical objects as we perceive them in space.
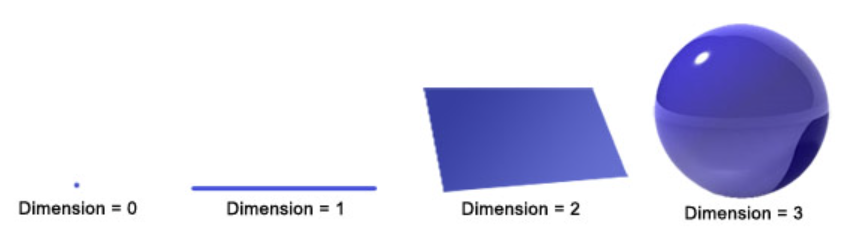
However, when it comes to dimensions beyond the third, we run into a cognitive limit. Visualizing a fourth spatial axis, one that is perpendicular to all three we already know, is beyond the capabilities of our spatial perception. As three-dimensional beings, we can infer the existence of higher dimensions mathematically or symbolically, but we cannot fully visualize them in the same way we understand length, width, and depth.
Do Fractals Have Dimensions?
Yes – And They’re Not Whole Numbers
The world of fractals is rich and varied, and different types are classified based on how they’re generated, what properties they exhibit, and where they appear (in math vs. nature).
For a shape to be considered a fractal, it must:
-
- Have detail at every scale (or self-similarity across multiple scales)
-
- Be too “complex” for its topological dimension
-
- Have a non-integer (fractional) dimension
Some examples of fractals and their key features.
| Type of Fractal | Key Feature | Example |
| Self-Similar | Identical repetition at all scales | Sierpiński Triangle |
| Self-Affine | Stretched/scaled versions at each level | Geological formations |
| Random (Stochastic) | Includes randomness | Clouds, terrain, lightning |
| Escape-Time | Based on how fast values “escape” | Mandelbrot Set |
| Iterated Function System | Built from geometric rules/transformations | Barnsley Fern |
| Strange Attractors | Chaotic systems in motion | Lorenz Attractor |
| Space-Filling Curves | Fills space without overlap | Hilbert Curve |
Now, when I say “fractal,” I want to be precise. The word “fractal” gets thrown around a lot, and understandably so, there are many kinds of fractals, from strictly self-similar ones like the Sierpiński triangle to chaotic boundary sets like the Mandelbrot. That variety is exactly why it’s often hard to pin down meaningful discussions online; people can be referring to entirely different phenomena using the same word.
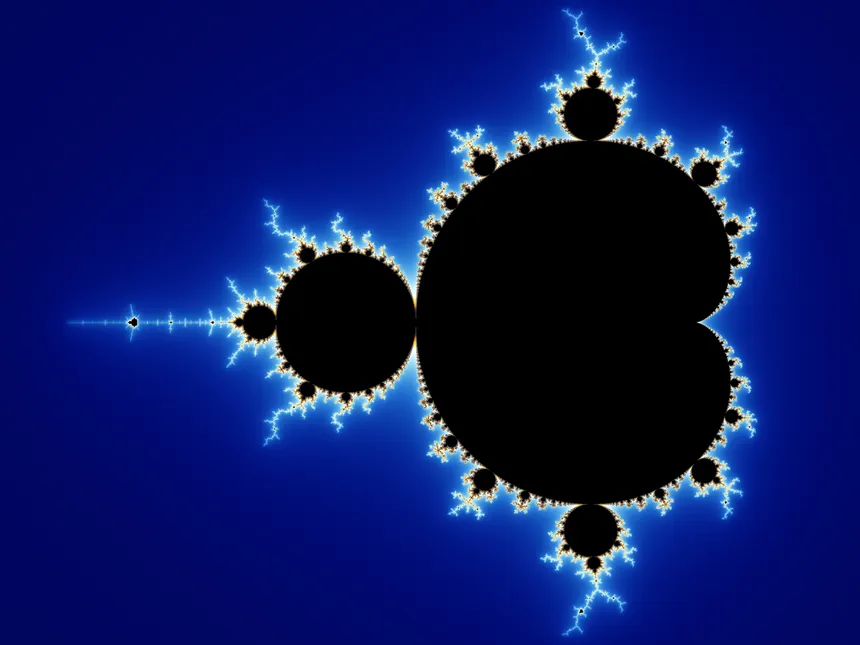
So in the context of my hypothesis, let me be specific: I’m referring to the Mandelbrot set as the conceptual model, not because I think we literally reside in it, but because it uniquely demonstrates how infinite, recursive structure can emerge from deceptively simple rules, and how dimensional boundaries can blur into deeper complexity.
What Is the Mandelbrot Set?
The Mandelbrot set, which is represented by the equation zn+1 = zn2 + c, is plotted on a two-dimensional complex plane, but its boundary has a fractal dimension between 1 and 2. How is this possible? Let’s recall that a circle is a simple two-dimensional shape that exists on a flat plane. The circle is considered two-dimensional because it encloses a finite, well-defined area with a smooth, closed boundary.
In contrast, the Mandelbrot set behaves very differently. Its boundary is infinitely complex and never “closes” in the smooth, finite way a circle does. No matter how far you zoom in, new layers of intricate structure continue to emerge. You could trace its edge forever, it never resolves into a simple, enclosed loop. In this way, the Mandelbrot set’s boundary defies traditional geometric closure, never quite fitting the classification of a true two-dimensional shape. Instead, it embodies an infinite unfolding within finite space. For more information on the Mandelbrot set click here.
What Is the Fractal Dimension Hypothesis?
I propose that our lived reality may occupy a fractal dimension between 3 and 4. We experience it as three-dimensional, but violations of linear space-time suggest there may be more going on than a clean, integer-based spatial framework. Instead, reality may be the projection or unfolding of a more intricate, higher-order fractal geometry. Within our bounded world, we still experience phenomena that hint at interaction with something beyond it. Things like:
-
- Ghost sightings and apparitions
-
- Mediumship and communicating with the dead
-
- Déjà vu
-
- Psychic intuition
-
- Past life memories
-
- Telepathy
-
- Near-death experiences
-
- Unexplained synchronicities
-
- Astral projection
These aren’t just strange occurrences, they’re violations of linear space-time. For instance, when a medium brings through a message from someone who has passed, alongside an accurate description of them that the medium could have never known from a memory or by other means. Or if a remote viewer sees a pyramid on Mars, they are interacting with information that bypasses space and time entirely.
To expand on this idea: imagine the bulbous center of the Mandelbrot set, the solid, stable region, analogous to our lived experience here on Earth. It’s where the values are bound, where things make sense, where time and space feel consistent. But as you move outward, approaching the edge of that central bulb, you enter the infinitely complex boundary. I see this as similar to what we perceive when we look out into the cosmos. No matter how far we peer into space, we never reach a true edge. There’s always more, more galaxies, more questions, more mystery.
If we can attribute the violations of space-time to the fourth dimension we cannot perceive but we intuit, I certainly feel like our world is constantly approaching the fourth dimension, just as the Mandelbrot set approaches two dimensions without ever fully becoming a true two-dimensional shape. It’s always unfolding toward that next level of complexity, but never fully actualizing it.
How is that possible unless consciousness has access to a higher-dimensional structure that we cannot fully perceive?
Just because we can’t see this dimension doesn’t mean it’s not there. After all, the simple formula behind the Mandelbrot set contains not just the solid region, but the boundary, the infinite detail, and infinity itself. It’s all encoded in one compact expression.
If we’re using the Mandelbrot set as a metaphor for reality, and we imagine ourselves existing within the bounded interior, it may seem paradoxical that we can access the infinitely complex boundary (or anything beyond it) without physically traversing that distance. But here’s the key: the boundary in a fractal like the Mandelbrot set isn’t spatially far away, it’s perceptually encoded. In fact, every point within the set is infinitely close to the boundary if you zoom deeply enough. Psychedelics and other plant medicines may function like a cognitive zoom lens, temporarily altering the brain’s filtering mechanisms and revealing patterns and dimensions that are normally hidden from ordinary perception.
The brain itself is a fractal system, with recursive structures in its neural architecture and activity patterns. Under psychedelics, the brain’s default mode network which usually keeps our experience grounded and predictable becomes disrupted. This allows for a flood of sensory, emotional, and symbolic information to surface. In that altered state, one may begin to perceive non-linear patterns, seemingly impossible geometries, or even entities that exist outside of time, phenomena that mirror what we find when zooming in on the Mandelbrot set’s fractal edge. From this perspective, we’re not being transported somewhere else; instead, psychedelics reveal that the deeper structure of reality and consciousness itself already exists at the fractal boundary between order and chaos.
So, while the boundary may seem “far” in abstract terms, it is actually embedded all around us — hidden behind the cognitive and perceptual structures we take for granted. Psychedelics don’t take us to another place; they remove the filters that keep us from seeing the deeper, more dimensional nature of where we already are.
| Mandelbrot Region | Analogy | Experience |
| Inside the Set | Physical Reality / Incarnation | Order, structure, “normal life” |
| Boundary of the Set | Bardo / Duat / Liminal Consciousness | Chaos, psychic phenomena, the veil |
| Outside the Set | Infinity / Source / Spirit | Formless, transcendent, ungraspable |
The Inside of the Mandelbrot Set
(Points that stay bounded under iteration)
Represents “Reality” as we know it
-
- This is the physical, material world.
-
- It’s predictable, structured, and navigable.
-
- Our daily experience: time flows forward, cause leads to effect, objects are stable, and the laws of physics are consistent.
-
- You could call this the realm of incarnation in flesh and form.
The Boundary of the Set
(Infinite detail, never repeats, chaotic yet not divergent)
Represents the Bardo, the Duat, the Astral Realm
-
- The in-between — not fully alive, not fully dead.
-
- It’s where consciousness detaches from the constraints of space-time, allowing for psychic phenomena, ghost encounters, mediumship, precognition, and symbolic realities.
-
- This is the domain where meaning and pattern still exist, but linear logic breaks down.
-
- It’s also where we might go during altered states, astral projection, or near-death experiences.
-
- It’s symbolically rich — like the Egyptian Duat or the Tibetan Bardo, it presents symbolic trials, lessons, and messages, often shaped by our perceptions and karmic patterns.
The Outside of the Set
(Points that escape to infinity)
Represents the Absolute or the Infinite
-
- Pure formlessness, beyond duality.
-
- Maybe Source, the void, or full spiritual dissolution.
-
- There’s no “you” here, no symbols, no narrative, no shape.
-
- It’s unknowable in the way that we can’t comprehend true infinity or timelessness.
What If Perceived Reality Exists at the Boundary, Not the Center?
The infinitely complex boundary is the edge where order and chaos collide. Every zoom reveals new, intricate patterns, where you can never truly find repetition, yet structure is still somehow present.
Could it be possible that that we’re navigating the razor-thin edge between the two? The human experience, dreams, paradoxes, quantum uncertainty, psychic impressions, synchronicities could all be coming from this fractal frontier of organized chaos.
From this perspective, we are the fractal boundary, the living, perceptual interface between the known and the infinite.
Why this also works:
-
- Inside the set: The realm of spirit, karma, and timelessness; potentially the akashic records, where all data is stored.
-
- The boundary: Emotion, evolution, entropy, choice, free will.
-
- Outside: The experience of losing all reference points, perhaps in death or enlightenment.
This duality is exactly what fractals and mysticism are all about. It mirrors the Hermetic axiom:
“As above, so below; as within, so without.”
In one sense, life is the safe center and death is the wild unknown. In another sense, spirit is the calm core, and incarnation is the chaotic surface.
It depends on which axis you view from, are we fractaling into form, or out of it?